As we have learned previously the area of a rectangle is equal to the width multiplied by the length. Since a rectangle has four sides and opposite sides are equal there is only two measures to work with, you just multiply them. When we are working with area in the real world most values are not round and easy to work with. When it comes to length when working on construction sites it is most often measured with a tape measure. In the United States we use the U.S. customary measurement system where length is measured in feet and inches. Most tape measures that measure inches break them into 1/16ths units. This almost ensures that every time you are determining area, you are working with fractions. You should get more relaxed with multiplying these values, it is definitely set to cross paths with you in the future. This selection of worksheets and lessons will help you find the area and perimeter of rectangles that contain measures with fractional sides.
Make sure to allow time for the example to sink in for students. I spent extra time working it for them.
I throw in some fraction operations here. They might be just the trick to spark some past material in students' brains.
I removed the rectangles to focus on the underlying core skill to this section. I will add rectangles in future quizzes.
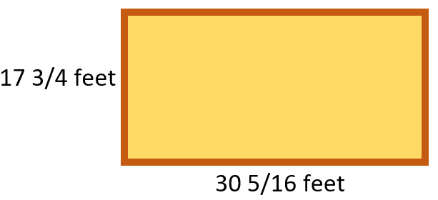
Find the area of the floor rug found below.

Step 1- Setup Problem
We are finding the area of a rectangle. The area of a rectangle is the length multiplied by the width. The length (longest side) in this case is 30 5/16 feet. The width (shorter measure) is 17 3/4. To setup the problem the answer will be the product of 30 5/16 x 17 3/4.
Step 2- Get All Measure to Share the Same Denominator.
We need to find a common denominator for our mixed numbers. In this case 16 would be the lowest common denominator, length is already in that denominator, so we will focus our attention on converting the denominator of the width (17 3/4). The conversion would result in restating the width as 17 12/16. That leaves us with the measures at: length = 30 5/16 feet and width = 17 12/16 feet.
Step 3 – Convert to Improper Fraction
We will convert the mixed numbers to improper fraction. This means that we multiply the whole number by the denominator. We then add that product to the numerator. Here is how to convert each mixed to an improper fraction:
Length: 30 5/16 = a) denominator product = 30 x 16 or 480. b) adding product to numerator = (480 + 5 = 485). The final improper fraction reveals as: 485/16
Width: 17 12/16 = a) denominator product = 17 x 16 or 272. b) adding product to numerator = (272 + 12 = 284). The final improper fraction reveals as: 284/16
Step 4 – Multiply the Improper Fractions
So, we would be left with (485/16) x (284/16). We would multiply the numerator by numerator (485 x 284 = 137,740) and the denominator by the denominator (16 x 16 = 256). When we put this final product together, we are left with 137,740/256 feet.
Step 5 – Reduce the Fraction
We would find the 256 goes into 137,740 - 538 times with a remainder 12. This means that our final answer would be 538 12/256. We can reduce the fraction even further to 538 3/64 feet.

As we begin to determine area with measures that include fraction, we will often come across an old skill that most of us could use a quick refresher on. In some cases you will be multiplying whole number and fractions, but more often than not you will be working with fractions that have different bottoms (denominators). Multiplication of unlike fractions with unlike denominators is considered difficult by many students, which shouldn't be the case at all. Let's try and understand how we can multiply fractions with unlike denominators.
Let us take an example of splitting a pizza between a group of people. One person grabs 2/3rds of the pizza, and now you are supposed to share the remainder of the pizza equally between two other individuals. What will the fraction be when divided between them?
There are a few simple steps that need to be considered here.
Make an expression by setting up the problem. In this case, the expression will be:
1/2 of 1/3 = 1/2 x 1/3 (Unlike Denominators)
The bottom numbers are different from each other. It can be a problem when you are adding or subtracting the fractions, but never when you are multiplying.
- Multiply the top numbers with the numerator
1 x 1 = 1 (numerator for your answer)
- Now multiply the denominators
2 x 3 = 6 (This will be your denominator)
- Now put your answers together which will be = 1/6